Ed Explores: Silver Rectangles

Before I start talking about silver rectangles, I’d like to introduce the first of what will hopefully be a number of articles. “Ed Explores” is simply me, looking at various topics that I find interesting and that also relate to geometric aspects of origami.

I’ve never been a great fan of math, myself, despite studying it in some shape or form to degree level. But geometry has always interested me: It’s something that can be seen in everyday objects as well as in nature. I’ll try to keep the math to a minimum, however, and where possible, explain concepts using illustrations.

Those of you who know me will also know that I really enjoy folding modular origami, particularly Sonobes and kusudamas. I find their geometry and symmetry fascinating, and it was this that led me to delve into the math behind them.

So, What’s a Silver Rectangle?

Before you ask, a silver rectangle is not simply one cut from tin foil! Most of you will already be familiar with silver rectangles, even though you may not be aware of it. Unless you live in North America, the chances are you’ll use paper in this shape. The ratio between the two sides of a silver rectangle is 1:1.414, or to be precise, 1:\(\sqrt{2}\). For most purposes 1:1.414 is close enough and is often referred to as A-ratio (as used in the A-series of paper sizes: A5, A4, A3, etc.). These sizes are defined in ISO216, which is an international paper standard.

Why the ratio 1:\(\sqrt{2}\)?

Why not use 1:2, or any other ratio for that matter? A4 paper measures 210 × 297 mm. If we multiply 210 mm by 1.414, we get 297 mm, which is the length of the longer side. Similarly, if we multiply 297 mm by 1.414, we get 420 mm, which is the length of the longer side of A3. What do you notice about these two sizes? A3 is exactly double the size of A4. 1:\(\sqrt{2}\) is the only ratio where this is true. If you double the size of any silver rectangle, you’ll always get a bigger silver rectangle with the same ratio. Conversely, if you halve a silver rectangle, you guessed it, you’ll get a smaller silver rectangle. The diagram below illustrates a range of paper sizes in the A-series.

A-series paper sizes.

The outermost edges represent an A0 sheet of paper. All smaller sizes can be made by repeatedly halving the previous size. To give you an idea of scale, A6 is commonly used for postcards (approximately 6″ × 4″). So, why is A4 commonly used for documents? As can be deduced from the diagram, it is one-sixteenth of A0. But why should this matter?

A0 is 841 × 1189 mm, which is very nearly one square meter. Paper weights are generally specified in grams per square meter (gsm or g/m²). An A0 sheet of 80 gsm paper weighs 80 g, and therefore, an A4 sheet of 80 gsm paper will weigh one-sixteenth of this (5 g). This relationship means that you can easily calculate the weight of a printed document and therefore how much the postage is likely to be. Currently, in the U.K., a standard letter can weigh up to 100 g, measure 24 × 16.5 cm and be one-half cm thick. This is equivalent to approximately 19 sheets of A4 80 gsm paper folded in half and enclosed in a C6 envelope. Note that B and C paper sizes supplement A sizes (see further reading).

Anyway, “let’s get back to origami,” I hear you cry. But, before we do, a word of warning: ISO 216 specifies tolerances for paper sizes. For paper between 150 mm and 600 mm in size (A4, A3 and A2), a tolerance of ±2 mm is acceptable. This means that a sheet of A4 copy paper could potentially be 2 mm larger or smaller in either direction, which differs considerably from our ratio of 1:1.414. So, if a silver rectangle is required for your model, this inaccuracy could cause problems. My advice would be to check your paper before you use it to fold. Copy paper isn’t usually that bad, and you can always adjust it a little, if need be. Note that by dividing a larger sheet, any error is halved each time. Using a worst-case scenario (±2 mm), A4 paper cut into 16 equally sized pieces (A7), should now have an error no greater than ±0.125 mm on each side.

Creating Silver Rectangles

You might be thinking, “it’s all well and good saying A-ratio paper is a silver rectangle, but what if this paper isn’t readily available?” Letter paper, as used in North America, measures 8½″ × 11″. This ratio is approximately 1:1.294, which is too far out from our 1:1.414. But, if you remove ¾” from the width, so your paper now measures 7 ¾″ × 11″ the ratio should be 1:1.419 … close enough! Note, this is calculated by either multiplying the shorter side or dividing the longer side by 1.414.

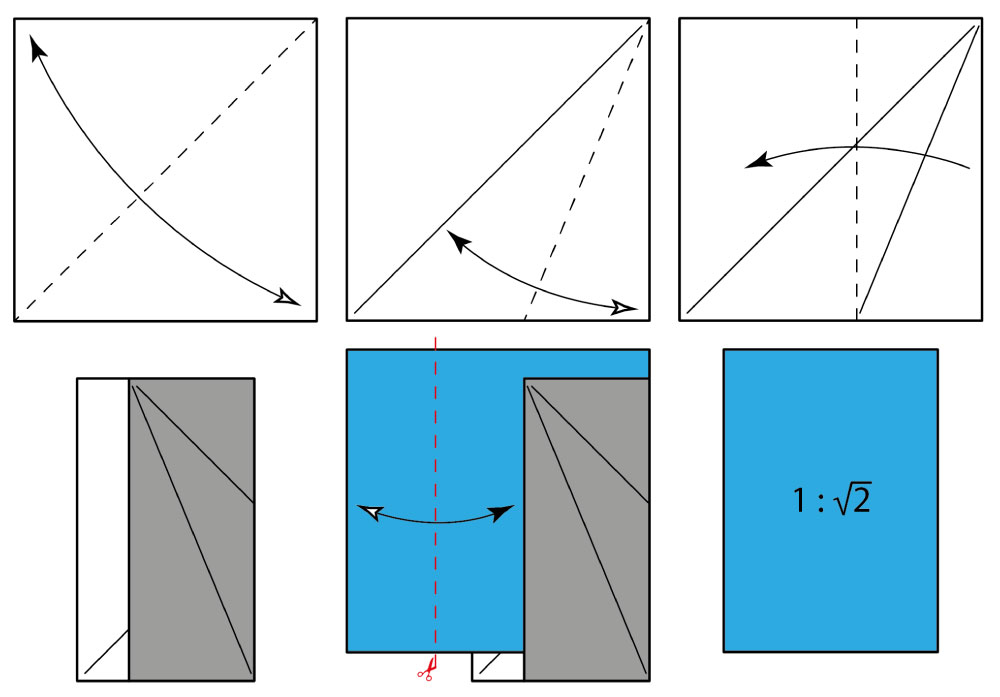
But what if you’re using squares (a regular starting point for most models). You can apply the same logic. Divide your square by 1.414 and cut off the leftover paper (which will have the ratio 1:2+\(\sqrt{2}\)). Alternatively, you can fold a template that can be used to create silver rectangles (see illustration below). Simply fold the template, insert a piece of the same sized paper and fold the raw edge to meet the raw edge of the template; then cut along the fold line. You may even be able to use the leftover piece for another model.

Folding and Using a Silver Rectangle Template.

Models that use Silver Rectangles

I guess you’re wondering why I’ve talked so much about silver rectangles yet not mentioned any models that use them? This is an area of origami that I feel should be explored further, especially considering the wide availability of A4 paper.

Models Folded from Silver Rectangles.

The photos above show some of my favorite models folded from silver rectangles. Left: Robert Foord’s Matryoshka Rhombic Dodecahedron (published in British Origami 317, Aug. 2019). Center: Nick Robinson’s A4 Rhombic Unit (this is actually constructed from 12 units, but it looks like a cube when viewed from this angle). Right: Francesco Mancini’s Kusudama UVWXYZ (a video by Tadashi Mori). I know there are further examples out there, but obviously, I can’t include them all in this list.

I’d also recommend the following books: British Origami Society Booklet 21, “The Silver Rectangle” by John Cunliffe (ISBN: 978-1515282860), “Brilliant Origami” by David Brill (ISBN: 0870408968) and “Mathematical Origami” by David Mitchell (ISBN: 978-1899618187).

Why a Silver Rectangle?

In the article “I Name This Shape” (British Origami 75, April 1979, p.12), John Cunliffe references a request in the Sunday Telegraph to name the 1:\(\sqrt{2}\) rectangle. Many suggestions were made, but the name proposed by lexicographers from the Oxford English Dictionary organization, “silver rectangle,” seems to have stuck with the origami community.

Interestingly, for mathematicians, “silver rectangle” refers to a slightly different rectangle that has a ratio of 1:1+\(\sqrt{2}\) or 1:2.414. Curiously, if you remove a square from a sheet of A-ratio paper, the leftover piece is 1:2.414, thus it follows that if you remove a square from the leftover piece, you get back to A-ratio. Interestingly, the ratio between the width and side length of a regular octagon, is also 1:1+\(\sqrt{2}\).